How to Rationalize the Denominator
To Rationalize a denominator sounds a little daunting, but if your student has learned the correct math vocabulary up until now, it is not difficult to break this statement down.
What does 'Rationalize the Denominator' mean?
When we 'rationalize the denominator' of a fraction, we change the denominator (which is a radical number) into a rational number. That's it.
How do we change from a radical to a rational number?
We are simply doing some math, above and below the line of the fraction (we are not changing its value) so we can remove a root from the denominator. The reason we do this is because for a fraction to be in its simplest form, it should not contain a root in the denominator.
It is absolutely okay for a root to appear in the numerator (above the line of a fraction)!
So, when a fraction has an Irrational Denominator - we have to 'fix' it.
Rationalize the Denominator - Worked Examples
I have two worked examples below to show how this process is done. The first, simply has a root as the denominator, and the second has to make use of a conjugate!
To successfully understand what is being done in these examples, please ensure you are comfortable with the rules of radicals.
Example 1
We have an irrational number (Root7) as the denominator of a fraction - we must 'rationalize' this.
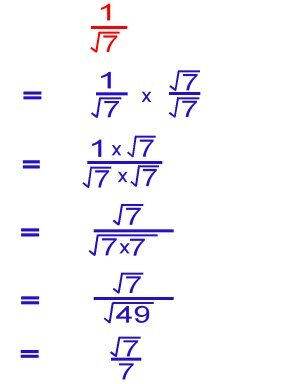 |
|
Example 2
We must remove the root 7 from the denominator.
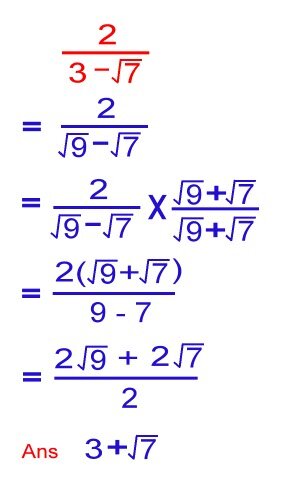 |
|
The first of these examples will not take your student long to grasp, however, the second, though they may understand quickly what must be done, it takes practice to be able to do it with little effort and brain power. Be sure your student practices this skill regularly! This is not a natural skill, there is nothing instinctual about rationalizing the denominator. The only way a student can be successful with this skill is to practice it as much as possible.
If you enjoyed the information presented here on how to rationalize the denominator, I encourage you to check out my other Basic Algebra material or explore the of what Printable Math Worksheets has to offer.
Keep In Touch!
You can send me a quick message, follow me on Facebook or why not join our community of like minded parents? Choose all the options so you don't miss any of the new material added to this site.